Saros, Spirals, and the Antikythera Project ~ Revelations from an Ancient Shipwreck
- Douglas MacDougal
- Feb 5, 2023
- 16 min read
Updated: Jul 21, 2024
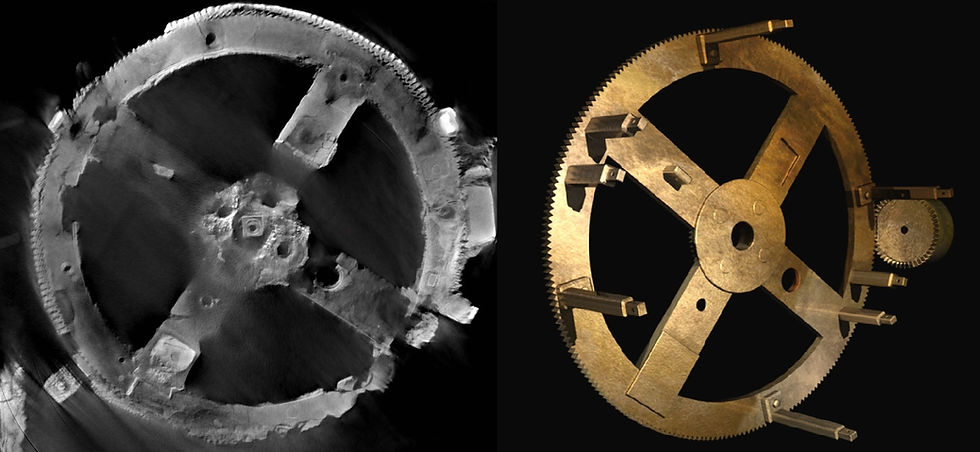
In 1901, sponge divers off the tiny Aegean island of Antikythera, Greece, discovered the remains of a Hellenistic era cargo ship which likely sank within a few years after 70 BCE. Among its recovered relics was a barnacle-encrusted mass. A protruding gear from it was discovered months later when the lump split apart. The find puzzled investigators for the better part of a century, but modern tomography and other techniques finally revealed the ‘Antikythera Mechanism’ to be a scientific marvel of the ancient world: a sophisticated mechanical computer of solar system movements offering a glimpse of the little-known period of pre-Ptolemaic planetary science [1; 2]. After 2,000 years under the Aegean/Ionian sea, the fragments of the Antikythera Mechanism are now exhibited at the National Archaeological Museum in Athens, Greece.
Centuries of Babylonian records showed Mesopotamian peoples that over long spans of time, the moon and planets return to their same positions in the sky, each as if turned by a great rotating wheel. Central to the ancient human need to find order in their universe, the cyclic nature of solar system bodies meant their behavior could be accurately predicted – a monumental turning point in mankind’s relationship to the cosmos. The wonder of celestial predictability made eclipses of the sun and moon particularly suitable for modeling with gears and wheels; an approach fully in line with the ‘craftsman’ ethic of the Hellenic philosophers. The Greek word exeligmos means ‘turning of a wheel’ [2], and investigators discovered to their amazement that the Antikythera mechanism, with its Saros and Exeligmos dials, could accurately foretell eclipses employing lunar cycles discovered in Babylon at least half a millennium before the device was made [3]. Thus, the device has added to the growing body of evidence that the genius of Babylonian numerical astronomy had been broadly disseminated throughout the Hellenistic world. New data and imaging techniques even in the last decade have confirmed that “its design was a highly ingenious fusion of ancient Babylonian and Greek mathematical astronomy.[4]”
The Clockwork Cosmos
The gearing on the marine-encrusted lumps from the sea took decades of work to decipher. Imaging technology allowed inspection of its metal innards, stimulating a minor cottage industry of academic research. Work is ongoing; papers are still being published. The more scientists have uncovered, the greater is their amazement at the sophistication of this ‘portable cosmos.’ It turns out that the Antikythera mechanism’s tight collection of interlocking gears, dials, and markings were “almost certainly” used not just for forecasting eclipses, but to predict the motions of all five planets known in ancient times, and even to mark other phenomena of interest such as the four-year cycle of the panhellenic games, the 19-year Metonic calendar, and (conjecturally) the 76-year Callippic cycle [1]. It must have been a visual marvel to behold: the front display of the device incorporated revolving pointers bearing little spheres representing the five planets making their apparent motions around the Earth, as shown in this reconstructed image of the front display of the mechanism [5].

An outermost depiction of the ‘sphere of the fixed stars’ was represented by symbols for the twelve zodiacal signs, then enclosed spherical shells for the five planets, Sun, and Moon, with the small globe for earth at the center.
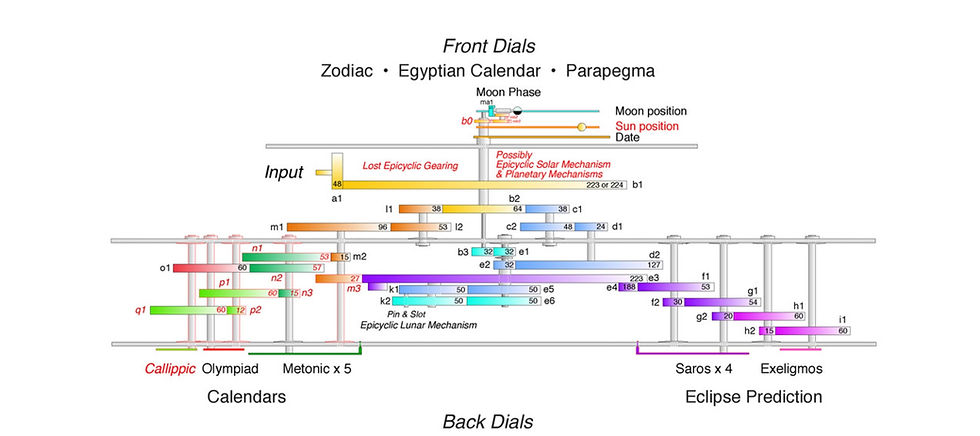
The image below is a schematic of the Antikythera’s gearing, both actual and reconstructed. The images above and below were created by Tony Freeth, an engineering professor with a solid mathematical background at University College London and a founding member of the Antikythera Mechanism Research Project, who was a pioneer in the study of the mechanism. Not only was the Antikythera Mechanism a wonder past comprehension of ancient ingenuity, but Freeth and Alexander Jones of NYU’s Institute for the Study of the Ancient World, with the earlier research help of Derek de Solla Price and many others, have created a marvel of ingenious historical reconstruction from its fragments [6].
Of course, the persistent question after discovery of the Antikythera Mechanism was, who could have made such a thing? No one without wide knowledge of Babylonian and Greek astronomical theory could have imagined this device. We’ll never likely know, but a tentative candidate for its design is Hipparchus of Rhodes, an exceptionally gifted observer and mathematician, the most important observational astronomer in antiquity, and one of the rare few who had the necessary knowledge to design such a device. (Almost all we know about him comes from Ptolemy, whose Almagest is admiringly peppered with references to his ideas and observations.) Tony Freeth, however, does not think that the Mechanism was made by Hipparchos, "in particular, because he did not have a planetary theory," and because birth/death dates "are probably too late."
Whoever may have designed it, Freeth and Jones have suggested that “[m]any aspects of the design of the Antikythera Mechanism suggest that it was essentially a mathematician’s instrument, “a brilliant mathematician’s creation.” It was probably designed for educational purposes. The design has a “purity of conception and an economy that is based on arithmetic cycles and the geometric theories current in the astronomy of its time.” It was a stunning technological achievement:
“For the mechanism to have worked, it must have been made to very close tolerances: in some parts it appears to have been constructed to accuracies of a few tenths of a millimeter. It is evidently the product of a sophisticated and mature engineering tradition and must surely have been preceded by a long history of development of similar devices.”
Moon Time
The moon was man’s clock: solar and lunar cycles and the rotation of the Earth were the primary units of calendar time in antiquity. Each month of the ancient Greek calendar was based on the new and full moon phases. The synodic month (the lunar cycle from new moon to new moon) was the fundamental time-keeping unit. The Olympic Games for example started on the 8th (or 9th) full moon after the winter solstice. Even though one tropical year (based on one full rotation of the sun) does not include an integer number of synodic months, the lunar cycle prevailed instead of the tropical year. It’s easy to see why. The moon is visible day and night; its phases change visibly and dramatically like a gigantic celestial clock. And at night, with patience, you can see it move against the background stars, just as you can see a clock hand slowly shift as you take the time to look.
Earlier from my September blog you’ll recall how the Babylonians discovered the subtle repeating characteristics of lunar motion: the synodic month (mentioned above) of about 29 ½ days, the anomalistic month (accounting for the moon’s variable motion) of about 27 ½ days, and the draconic month (the moon’s travel time from one node to the same node) of about 27 ⅓ days. They also became aware early on that solar and lunar eclipses can only occur in the vicinity of the lunar nodes, when the moon’s orbit intersects the ecliptic, so the sun, earth, and moon are lined up. Through long observation the Babylonians discovered that 223 synodic months = 239 anomalistic months = 242 draconic months. The 223 lunar months became known (in more modern parlance) as the Saros cycle, “a surprising arithmetic resonance between three orbital periods of the Moon,” in Freeth’s words. Tripling the Saros yielded the exeligmos cycle of about 54.1 years. The point of the Exeligmos Dial on the Antikythera Mechanism is to adjust the eclipse times by 8 hours after each Saros Cycle. Plain evidence of these periods has been found or inferred on the remnants of Antikythera Mechanism.
Spirals
The designer of the Antikythera mechanism incorporated a most clever eclipse prediction device on the back face of it. The mechanism relied on inscribed text to represent the predicted events in words, while a gear work-driven pointer functioned to tell the approximate date for each prediction on the dial. The largest ‘chariot wheel’ gear (shown above) had 223 teeth, corresponding to the Saros cycle of lunar months; this was connected to a hand crank. (The gear at the back, e3, had 223 teeth; the gear at the front, b1, probably had 224 teeth.) I’ll not go into the details here about what archaeologists, astronomers, Assyriologists, mathematicians, engineers, technicians, linguists, classicists, epigraphers, videographers, and all other manner of scholars have learned about the construction and functioning of the Antikythera mechanism. Suffice to say, the effort to reconstruct and decipher it has been a remarkable demonstration of cross-disciplinary scholarship. I’ll focus here only on the “Saros spiral,” a marvelously ingenious feature of the device that was the main interpretive vehicle for eclipse prediction.
Beneath the removable back cover, there were two dials each in the form of spirals, each with a pointer mechanism. The top dial showed a 19-year Metonic calendar, evenly divided into 235 lunar months [1, 1.4; 3]. (The Metonic cycle results from the close equality of 19 years to 235 lunar months. It represents the return to the same phase of the Moon on the same date of the year. After the cycle, the Sun, Moon, and Earth are back in nearly the same relative orientations.) Inside the Metonic dial was a smaller dial, showing the 4-year panhellenic games cycle and (conjecturally) a dial showing the 76-year Callippic cycle (which is four Metonic cycles minus one day).
The bottom dial, our focus here, was the 223-month eclipse prediction dial, based on the Saros cycle. This dial included glyphs with information about future eclipse possibilities, including day and expected time of the eclipse. If the pointer on the spiral made 4 complete terms, it would cover 223 lunar months’ worth of motion depicted along the length of the spiral – the whole Saros eclipse cycle [7]. After each 223-month run, the pointer would have to be reset to the beginning.
Inside the Saros dial was a small Exeligmos dial designed to adjust the eclipse times for successive whole revolutions of the Saros dial [1, 2]. The Exeligmos dial was divided into three equal sectors, inscribed empty, 8, and 16, representing the number of hours that were to be added to the eclipse times indicated on the Saros dial. This was necessary because the mean length of 223 lunar months is about 8 hours more than a whole number of days, and the exeligmos cycle incorporates three Saros cycles; the little wheel was thus an error-correcting device to accommodate and correct for the 1/3 day ‘error’ inherent in each Saros (hence, no correction on the first run; add 8 hours on the second, and add 16 on the third). (If this is unclear to you, you might wish to check my September post here which explains the exeligmos cycle in more detail.)
The conceptual heart of all the wheels and gears was the elegant form of the spiral. Spirals are plentiful in mathematics; they are typically beautiful. The spirals on the mechanism were obviously carefully ‘turned’ in some way to achieve a high level of accuracy in the spacing so that everything fit, and yet allow room for the spiral’s glyphs discussed below. Spiraling data on a small two-dimensional surface was certainly a marvelously economical way to depict each of the 223 lunar months of the Saros cycle. But it had to be near-perfect to operate as a calculating machine. How could they have fashioned the evenly spaced cells in each row of the spiral so as to make the outcome be 223 perfect cells at the end of the spiral? Try it with paper and compass – it is not easy!
To better understand the tricky task of creating such a spiral with its cells spaced just so, I briefly ‘sketched’ one using mathematical software to see how the fit of cells would occur consistent with the design of the Antikythera Mechanism. The model was constrained by four conditions: It must be (i) an ordinary spiral, or "joined semi-circles" (as Freeth has described them) with constant spacing between the tracks (ii) encompassing four turns (iii) accommodating just 223 cells (iv) all of which must be of equal angular separation throughout the length of the spiral (but also of necessity they must be able to vary in size as one progressed through the spiral).
Fascinatingly, Tony Freeth told me he believes "the Antikythera Mechanism was used as its own “dividing engine” for marking the scale divisions on the Saros Dial. "For each scale division, the Mechanism was turned forwards by a synodic month — as indicated by the lunar and solar pointers on the Front Dial — then the next scale division was marked."
As you can see in the image below, I didn’t include the pointer, Exeligmos dial, or embellishments: it’s the spiral form with boxes as stand-ins for the Antikythera Mechanism’s cells’ size and positioning. As with the mechanism, the inner track is the starting point of the spiral. The pointer on the mechanism moved clockwise, month by lunar month, like the arm of a (slow, hand-turned) record player, moving the pointer’s ‘needle’ in the groove (the red track here; black on the last image below) outward along the spiral, pointing to the applicable cell. Because 223 is a prime number, and not evenly divisible by anything, the ‘cells’ in the picture are slightly offset from each other, row by row, as they appear on the mechanism.

Now that you understand the bare form of the spiral, we’ll look briefly at the all-important mysterious markings, or glyphs, on the Saros dial. As you read about the glyphs, look closely at Tony Freeth’s reconstructed image below of the Saros dial and inscriptions [8] and see if you can pick out the meanings of some of them.
Glyphs
Each lunar month on the Saros dial was shown as a small cell along the path of the spiral. Cells for months within which no eclipse would occur were left blank. If a cell did correspond to a particular lunar eclipse possibility, it was inscribed small capital Greek letters and numbers (a ‘glyph’) describing the type of eclipse and when it would occur. For example, the first letter of the Greek word Selene for moon is sigma, so a Σ in the cell indicated a lunar eclipse. The first letter of the Greek word for sun is Helios, so Η in the cell indicated a solar eclipse [9].
Hours were indicated by a peculiar anchor-like symbol symbol (a ligature of omega and rho, according to Freeth) depicting the first two letters of the word ‘ΩΡΑ’, hora, the Greek word for hour, followed by a number indicating the time of the day or night. The rest of the letters represent ancient Greek numbers. For lunar eclipses, eclipse times were given in numerals from 1 to 12, which was presumptively during nighttime unless followed by an abbreviation using the Greek letters H and M, from the first two letters of the Greek word hemeras meaning “of day.” For solar eclipses, daytime hours were presumed unless the hour was prefixed by the first letters Y and N from the Greek word nyktos for night.
About 50 or 51 of the 223 lunar month divisions of the lower back Saros dial were inscribed with a glyph. When the pointer reached a division with glyphs, the user was advised that, on that month a solar (H) or lunar (Σ) eclipse might take place – an eclipse possibility – reaching its maximum at a particular equinoctial hour. The index letter or letters referred to inscriptions around the dials on the back plate and gave more details about the eclipse [6].
Here is Tony Freeth’s impressive reconstruction of the Saros dial on the Antikythera Mechanism, with some of the glyphs visible.

The spacing of solar and lunar eclipse events on the dial follows the typical 5- and 6-month spacing between eclipse possibilities known from Assyrian and Babylonian letters and reports [10]. I encourage you to pore over Freeth’s image at leisure, look at the glyphs, and lose yourself in a unique epoch of time.
Epoch
What is an epoch? Everything in the heavens is in motion, so there needs to be an anchor point, a start date fixing celestial positions and serving as the base frame of reference for future calculations and observations. An epoch is a reference point for the specification of any celestial coordinates. For example, the epoch used by Claudius Ptolemy in his Almagest for all his tables of the mean motions of the planets was February 26, 747 BCE, during the reign of King Nabonassar. From that base reference date, chosen because data from Babylonian tablets was available (transmitted to him through Hipparchus) his tables allowed one to mathematically spin the planets forward (or backward) and tell where they would be in the sky at any other selected date [11]. The standard epoch for modern astronomical observations is a bit more recent! It is noon, January 1, 2000 (usually cited as J2000.0).
What was the epoch for the Antikythera Mechanism’s gearing? Though not fully settled, some notable commentators have concluded that the mechanism is best fitted for an epoch of 28 April 205 BCE [13], the day of the new moon that month (and lunar perigee). It was the first full moon after that year’s spring equinox which fell on March 24th. It has also been suggested that the designer of the mechanism “was constrained in that the Saros epoch should coincide as closely as was practical with the moon at its apogee at full moon.” If we check the ancient lunar cycles, we find that the next full moon after epoch, on May 12th, was within a day of lunar apogee on May 13th [14].
April 28th, though, was not likely the starting position of the Saros pointer. It has been thoughtfully argued that the pointer at Cell 1 of the Saros dial was set on the lunar month beginning on April 29th, the day after epoch. April 29th would mark the time of first visibility of the moon, the consequential beginning of the month in ancient (and some modern) calendars. According to Freeth, "The months on the Antikythera Mechanism start at the first visibility of the new Moon crescent. This is why Sigma always precedes Eta in each month."
Now imagine you are somewhere in the Mediterranean in the month of May of 205 BCE. You want to see what is coming, moon-wise. See if you can count the lunar months clockwise from the beginning of the cells in the Freeth image and ‘predict’ the lunar eclipses of the next year and a half. Try to identify – or approximately identify – in the Saros dial’s cells these actual lunar eclipses of BCE calendar dates: June 11, 205, December 5, 205, May 31, 204, and November 25, 204 [15]. If so, you will have taken the first steps in using the Antikythera Mechanism for its intended purpose!
NOTES:
I am grateful to Professor Tony Freeth for his thoughtful review of this article and helpful corrections and comments.
[1] The discussion and quotations that follow on the ‘cosmological’ interpretation of the Antikythera Mechanism and its mathematically ingenious gearing to include all of the planets are drawn from Freeth, Tony and Alexander Jones. “The Cosmos in the Antikythera Mechanism.” Institute for the Study of the Ancient World (ISAW) Papers 4 (February 2012). http://dlib.nyu.edu/awdl/isaw/isaw-papers/4/. http://doi.org/2333.1/xgxd26r7. The purpose of the paper was to show how the mechanism, properly interpreted revealed the whole known cosmos in a single, incredible machine: “The Antikythera Mechanism is an astronomical calculating machine that predicted phenomena involving the Sun, Moon, stars and probably the planets—the latter being the focus of considerable debate and the subject of much of this current study. Our conclusion in this study is that the Antikythera Mechanism almost certainly calculated the motions of all five planets known in ancient times.”
[2] Jones, Alexander. 2007. A Portable Cosmos: Revealing the Antikythera Mechanism, Scientific Wonder of the Ancient World. Oxford: Oxford University Press. Antiquities scholar Jones at the Institute for the Study of the Ancient World at NYU has done an excellent job in his book of clearly describing in lay terms the mechanism, its function, and its historical and astronomical context.
[3] Freeth, Tony, et al. “Decoding the Ancient Greek Astronomical Calculator Known as the Antikythera Mechanism.” Nature Letters, 444 (November 30, 2006): 587-591. https://doi.org:10.1038/nature05357.
[4] Freeth, Tony. “Eclipse Prediction on the Ancient Greek Astronomical Calculating Machine Known as the Antikythera Mechanism.” PLoS ONE 9, no. 7 (July 2014): 1-15. e103275. https://journals.plos.org/plosone/article/file?id=10.1371/journal.pone.0103275&type=printable. https://doi:10.1371/journal.pone.0103275.
[5] Image from [1]. Unless otherwise credited, all photographic images in this article are reproduced courtesy of the Institute for the Study of the Ancient World, consistent with their Creative Commons Attribution License: Creative Commons — Attribution 4.0 International — CC BY 4.0. See Freeth's updated model: Tony, et al. “A Model of the Cosmos in the ancient Greek Antikythera Mechanism.” Nature Scientific Reports11:5821 (March 2021). https://www.nature.com/articles/s41598-021-84310-w. https://doi.org/10.1038/s41598-021-84310-w. Freeth, Tony.“Revising the Eclipse Prediction Scheme in The Antikythera Mechanism.” Palgrave Communications 5, no. 7 (2019). https://doi.org/10.1057/s41599-018-0210-9.
[6] One of the notable pioneers in the field was Derek de Solla Price. See e.g., Price, D. de Solla. “Gears from the Greeks: The Antikythera Mechanism — A Calendar Computer from ca. 80 BC.” Trans. Am. Phil. Soc. New Ser. 64, (1974): 1-70; and Jones, Alexander, "Like Opening a Pyramid and Finding an Atomic Bomb": Derek de Solla Price and the Antikythera Mechanism.” Proceedings of the American Philosophical Society 162, no. 3 (September 2018): 259-294. https://www.jstor.org/stable/45211597.
[7] From Freeth’s 2006 paper in [3]: “In the CT of fragment B we find a new feature that explains why the dial is a spiral: a ‘pointer-follower’ device … travelled around the spiral groove to indicate which month (across the five turns of the scale) should be read. From our CT data of the 48 scale divisions observed in fragments A, E and F, we establish 223 divisions in the four-turn spiral on the lower back dial, the spiral starting at the bottom of the dial. This is the Saros eclipse cycle, whose number is on the back door inscription. The 54-year Exeligmos cycle of three Saros cycles is shown on the lower subsidiary dial.” As to the pointer, he added (p. 589): “The pin was constrained to follow the groove between the spiral scales… causing the device to slide along the month pointer to indicate which ring on the spiral scale specified the month.” Hence it worked rather like a record player arm works in our era.
[8] Image reproduced courtesy of Tony Freeth’s Creative Commons Attribution License: Creative Commons — Attribution 4.0 International — CC BY 4.0.
[9] The glyphs and inscriptions are exceedingly minute: “Even by the standards of this period [2nd century BC], when stone inscriptions with letter height about 5 mm were not uncommon, the lettering on the Mechanism is tiny, with the letter height ranging from about 2.7 mm in the "parapegma" [the star calendar] inscription down to about 1.2 mm (i.e. smaller than modern 4 point type) in the inscriptions on the back spiral dials” [1, 2.4.2].
[10] See Steele, John M. “Eclipse Prediction in Mesopotamia.” Arch. Hist. Exact Sci. 54 (2000): 421–454. “Due to the different lengths of the synodic and dracontic months, the lunar node recedes in longitude by about 1;34◦ [1.57⁰] per month. During this same month, the sun on average travels about 29;6◦ [29.1⁰] forward in longitude. Therefore, the difference in longitude between the node and the sun (or the Earth’s shadow) at syzygy increases by roughly 30;40◦ [30.67⁰] per month. If we assume that eclipses do not occur in consecutive months, as it is apparent that the Babylonian astronomers did, it is possible to define an “eclipse possibility” as the syzygy at which the Earth’s shadow or the sun is closest to the node every time it passes by that node. The average interval between successive eclipse possibilities is equal to about 5;52,7,44 [5.87] months. Of course, this does not imply that eclipses possibilities occur every 5;52,7,44 months, for then moon and sun would not be at syzygy, but rather that eclipses occur every six months, with a five month interval every now and again.” Id. at 423 (numbers in brackets are my conversions from sexagesimal to decimal notation). Note: to check, if you divide 180 degrees by 30.67 degrees per month, you get 5.87 months.
[11] Because the motions of planets vary with precession and other gravitational perturbations from the whole panoply of planets, moons, and asteroids, orbital elements for one epoch are valid only for limited periods of time, depending on the level of accuracy needed.
[12] For an interesting and thorough analysis of the start-date issue from a classicist and epigraphist, see Iversen, Paul A. “The Calendar on the Antikythera Mechanism and the Corinthian Family of Calendars.” Hesperia: The Journal of the American School of Classical Studies at Athens 86, no. 1 (January-March 2017): 129-203.https://www.jstor.org/stable/10.2972/hesperia.86.1.0129. https://doi.org/10.2972/hesperia.86.1.0129. See also Seiradakis, J.H., and M. G. Edmunds. “Our Current Knowledge of the Antikythera Mechanism.” Nature Astronomy, no. 2 (January 2018): 35-42. https://doi.org/10.1038/s41550-017-0347-2.
[13] The discussion and quotes that follow draw on Iverson in [12]. On the topic of the mechanism’s epoch, see also Carman, Christián C., and James Evans. “On The Epoch of The Antikythera Mechanism and its Eclipse Predictor.” Archive for History of Exact Sciences 68, no. 6 (November 2014): 693-774. https://www.jstor.org/stable/24569560. Curiously, Carman and Evans found that the eclipse predictor would fit best if the full moon of month 1 of the Saros dial corresponds to May 12, 205 BCE, with the Exeligmos dial set at 0.
[14] The software used for these determinations by Alexander Jones, Paul Iversen, and perhaps others, and by me to check them, was Alcyone: http://www.alcyone-ephemeris.info/plsv_short_description.html. See especially, Jones, Alexander. “The Epoch Dates of the Antikythera Mechanism (With an Appendix on its Authenticity).” Institute for the Study of the Ancient World Papers 17, (2020). http://dlib.nyu.edu/awdl/isaw/isaw-papers/17/.
[15] From https://eclipse.gsfc.nasa.gov/SEpubs/5MCLE.html. Remember that the calendar dates in the literature are one year further back than the astronomical dates, so that 204 BCE is – 203 for software & many web applications, including NASA. Eclipse guru Fred Espanek’s explanation is here: https://eclipse.gsfc.nasa.gov/SEhelp/calendar.html and here https://eclipse.gsfc.nasa.gov/SEhelp/dates.html.


Comments